

 |
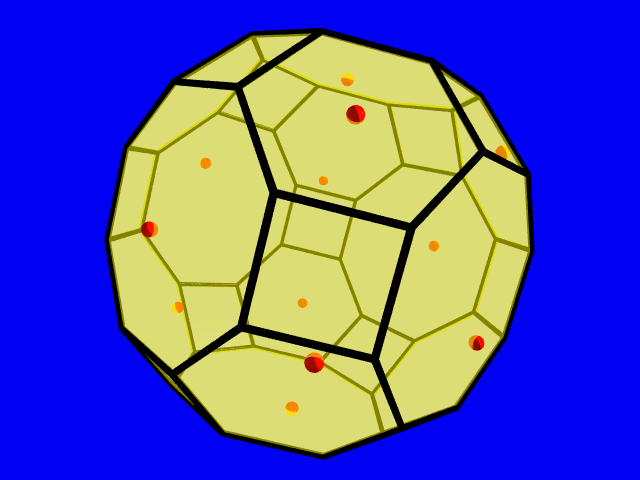
Densest lattice packing of a truncated cubeoctahedron
The truncated cubeoctahedron (sometimes called great rhombicubeoctahedron) has 48 vertices, 72 edges and 26 facets, 6 octagons, 8 hexagons and 12 squares. It is one of the thirteen Archimedean solids and its dual is called disdyakis dodecahedron. Maybe the first presentation of this polytope can be found in the revised version of Albrecht Dürer's Underweysung der Messung (around 1538).
The density of a densest lattice packing was calculated with the algorithm of Betke and Henk. The density is equal to 0.8493..., and the 12 points in the picture show the lattice points of a critical lattice lying in the boundary.
Model produced with: JavaView v2.00.a11
| Keywords | lattice packings; polytopes; packings; critical lattice; truncated cubeoctahedron | |
| MSC-2000 Classification | 52C17 (11H31) | |
| Zentralblatt No. | 01683004 |
Gif-file was produced by Povray 3.02
Submitted: Thu Feb 1 16:41:52 CET 2001.
Accepted: Fri Apr 27 14:11:54 CET 2001.
University of Magdeburg
Department of Mathematics
Universitätsplatz 2
D-39106 Magdeburg
henk@mail.math.uni-magdeburg.de
http://www.math.uni-magdeburg.de/~henk