A vertex decomposable 3-ball with a knotted spanning arc consisting of 4 edges

Author

Description

Hachimori and Ziegler [1] proved that a triangulation of a 3-ball which contains a knotted spanning arc consisting of at most 3 edges is not vertex decomposable. The following example (constructed along the ideas of Hachimori and Ziegler [1], yet considerably smaller) shows that 3 is the best possible upper bound for the number of edges.

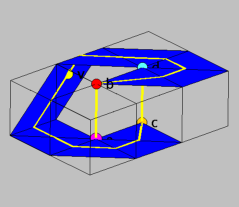
Let C₁ be the following triangulation of a pile of 3*2*1 cubes: Each cube is split into 6 tetrahedra, and the tetrahedra are all grouped around one of the diagonal axes of the cube. Furthermore let a denote the pink, b the red, c the orange and d the light blue vertex. For a face F and a vertex x let x*F denote the cone over F with apex x. Then C := C₁ union (v*(blue faces)), where v is a vertex not contained in C₁, is a 3-ball with the knotted spanning arc ab-bv-vc-cd. Since we add v*(blue faces) to C₁, the edges bv and vc actually exist. The edges ab and cd are already contained in C₁. To visualize the knotted arc, one may imagine it as following the yellow line. C is vertex decomposable: One can take v as the first shedding vertex.

Figure 1 depicts the pile of cubes and the faces needed for the construction are colored blue. The yellow line (including the yellow vertex v) visualizes the knotted arc. To obtain the example (vert_dec_3-ball_with_knotted_arc.jvx) one has to compleat the construction by adding v*(blue faces).

The master file vert_dec_3-ball_with_knotted_arc.jvx contains two geometries: The facets of the 3-ball (encoded as their vertex sets) and the knotted spanning arc (encoded as the vertex sets of its edges). In the master file point a corresponds to 15, b to 14, c to 9, d to 8 and v to 24. (If needed, check the applet file for the indices of the other vertices.) As shedding vertices one may use 24, 22, 23, 20, 18, 19, 21, 16, 17, 14, 12, 13, 15, 10, 4, 5, 11, 9, 0, 1, 3, 2, 6, 7 and 8 in this order.

References

1. M. Hachimori and G. M. Ziegler: Decomposition of simplicial balls and spheres with knots consisting of few edges, Mathematische Zeitschrift 235 (2000), 159-171.

Files

• Master File: vert_dec_3-ball_with_knotted_arc.jvx
• Applet File: vert_dec_3-ball_with_knotted_arc_Applet.jvx
• Preview: vert_dec_3-ball_with_knotted_arc_Preview.gif
• Original File (private file format): vert_dec_3-ball_with_knotted_arc.topaz

Submission information

Submitted: Tue May 8 20:14:13 CEST 2001.
Revised: Fri Jun 14 18:24:12 CEST 2002.
Accepted: Wed Nov 20 15:58:01 CET 2002.

Author's Address

TU Berlin
Fakultät 2, Institut für Mathematik, MA 6-2
Strasse des 17. Juni 136
10623 Berlin, Germany
witte@math.tu-berlin.de